Examples

This example simulates a theoretical cell with SiO2 + poly-Si contacts on both sides. It showcases the detailed full drift-diffusion modeling options available in the 1D solver of Quokka3. It is shown how to account for the influence of the poly-Si properties on the band-bending in the c-Si bulk, and thereby on the recombination properties. It also shows how a tunneling oxide can be considered explicitely via a direct tunneling model.

On the front side the n-type poly-Si layer is considered via a transport layer of type "metal-like". This means that a value for the workfunction is set, which defines the constant Fermi-Level in this layer, meaning there is no band-bending within this layer (like it would the case for metal). The barrier-height is not calculated from the workfunction difference to the c-Si absorber, which may be inaccurate due to neglecting barrier-lowering effects and band-bending in the poly-Si layer, but rather set to an empirical value. Also the thin oxide is considered explicetly as an insulator between the transport layer and the c-Si near-surface, via a direct tunneling model.

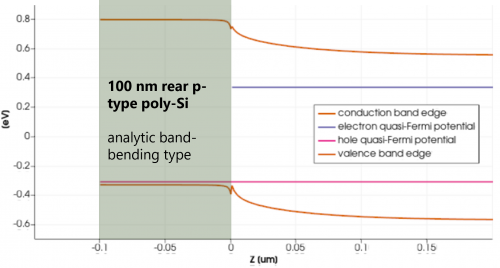
On the rear side p-type poly-Si layer a different approach is used. Here the transport layer is of type "analytic band-bending", which considers also band-bending in the poly-Si layer via an analytical model. This model requires definition of some material properties (band-gap / density of states / doping / activation energy), which in this example are assumed to be the same as c-Si. The model is valid if the layer's thickness is larger than its Debye length (i.e. band-bending relaxes within the layer thickness), and if the doping is sufficient to neglect any high-injection effects. This gives a physical more complete model of band-bending, and thereby also the influence on recombination via more correct prediction of carrier densities at the interface. Downside is that the analytical band-bending model is not compatible yet with explicitely resolving the oxide layer via a direct tunneling model.